Abstract: In this article, a novel infinitely smooth polyharmonic radial basis function (PRBF) collocation
method for solving elliptic partial differential equations (PDEs) is presented. The PRBF with
natural logarithm is a piecewise smooth function in the conventional radial basis function collocation
method for solving governing equations. We converted the piecewise smooth PRBF into an
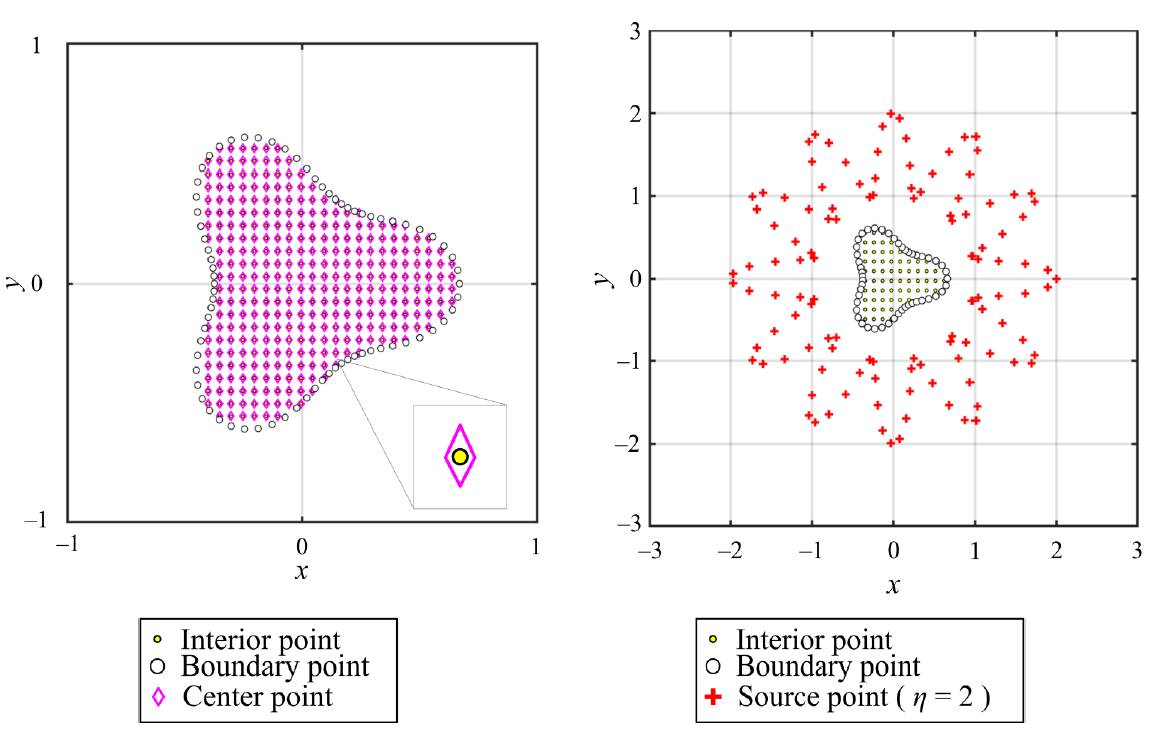
infinitely smooth PRBF using source points collocated outside the domain to ensure that the radial
distance was always greater than zero to avoid the singularity of the conventional PRBF. Accordingly,
the PRBF and its derivatives in the governing PDEs were always continuous. The seismic
wave propagation problem, groundwater flow problem, unsaturated flow problem, and groundwater
contamination problem were investigated to reveal the robustness of the proposed PRBF.
Comparisons of the conventional PRBF with the proposed method were carried out as well. The
results illustrate that the proposed approach could provide more accurate solutions for solving
PDEs than the conventional PRBF, even with the optimal order. Furthermore, we also demonstrated
that techniques designed to deal with the singularity in the original piecewise smooth PRBF are no
longer required.
Keywords: partial differential equations; polyharmonic; radial basis function; source point; collocation
method
https://www.mdpi.com/2227-7390/9/13/1535/pdf
https://www.mdpi.com/2227-7390/9/13/1535